
A specific example of a simple harmonic oscillator is the vibration of a mass attached to a vertical spring, the other end of which is fixed in a ceiling. An open question is to quantitatively explain how self-propulsion particles with directional memory diffuse in such a medium.
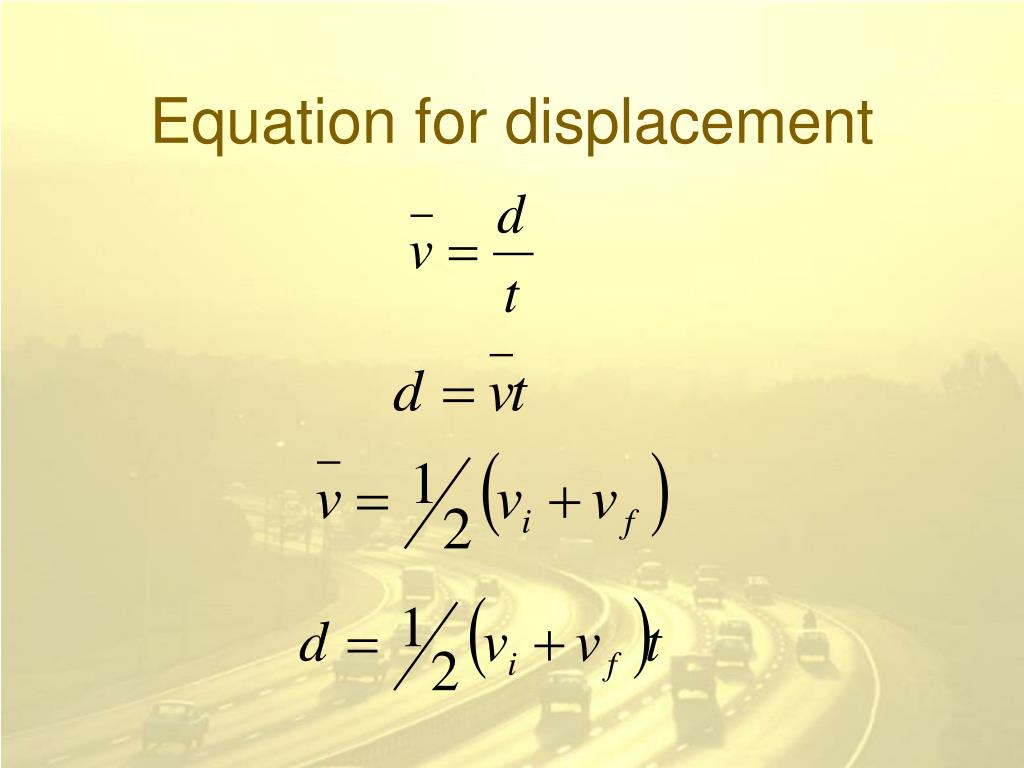
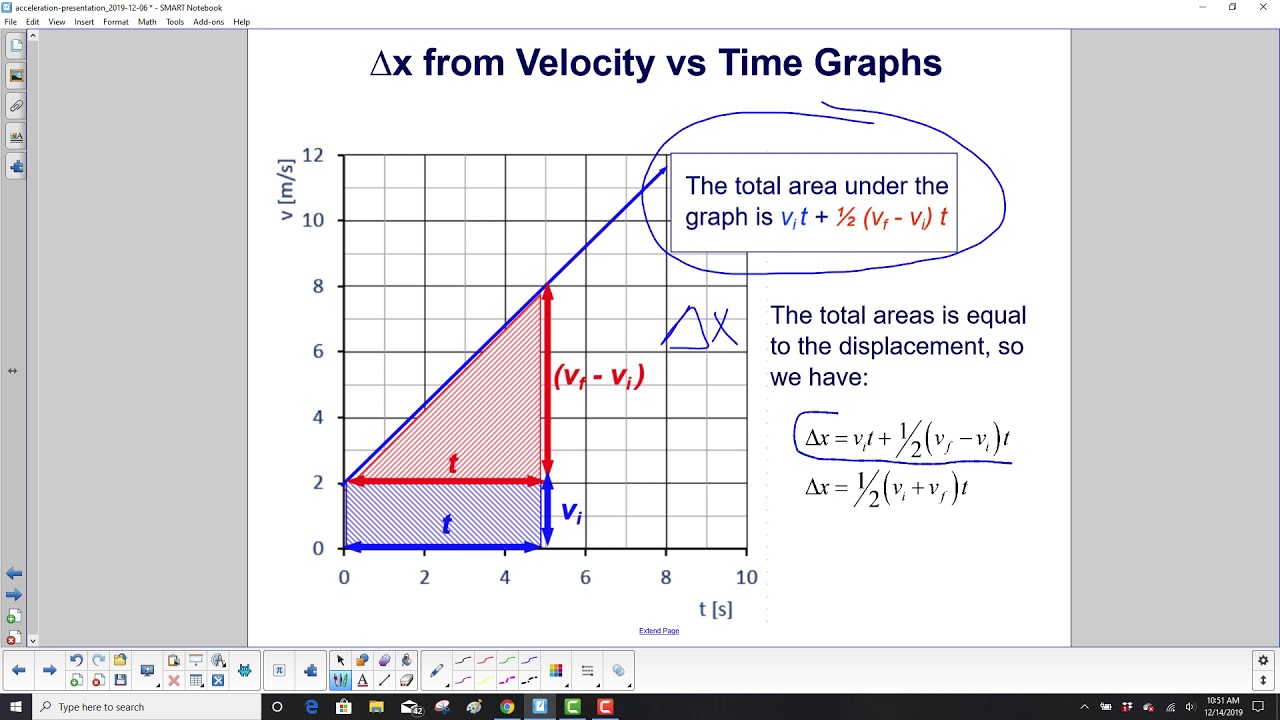
This is just a special case (a, equals, 0,a. In a viscoelastic environment, the diffusion of a particle becomes non-Markovian due to the memory effect. The distance traveled, however, is the total length of the path taken between the two marks. That is, F kx, where F is the force, x is the displacement, and k is a constant. svt where s,s is the displacement, v,v the (constant) velocity and t,t the time over which the motion occurred. The displacement is simply the difference in the position of the two marks and is independent of the path taken when traveling between the two marks. One way to think about this is to assume you marked the start of the motion and the end of the motion. In kinematics we nearly always deal with displacement and magnitude of displacement and almost never with distance traveled. In this text the upper case Greek letter (delta) always means change in whatever quantity follows it thus, x means change in position. In this case her displacement would be + 2 m +2 \text 1 5 0 m 150, start text, space, m, end text. Displacement is the change in position of an object: x xf xo, where x is displacement, xf is the final position, and x0 is the initial position.
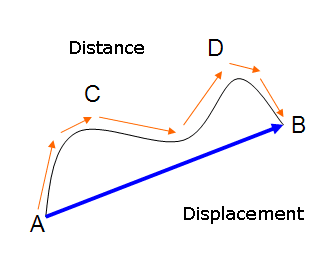
For example, the professor could pace back and forth many times, perhaps walking a distance of 150 meters during a lecture, yet still end up only two meters to the right of her starting point. Work Formula W Work done F Magnitude of the force applied d Magnitude of the displacement in the direction of the force. By magnitude, we mean the size of the displacement without regard to its direction (i.e., just a number with a unit). People often forget that the distance traveled can be greater than the magnitude of the displacement.


 0 kommentar(er)
0 kommentar(er)
